This article appeared in the March 19 issue of Executive Intelligence Review.

Bankers' math vs. human math: Do you know how to count?

by Dennis Small

The following is a slightly edited transcript of the speech given by Dennis Small on Feb. 14, 1999 at the Presidents' Day conference of the Schiller Institute and International Caucus of Labor Committees (ICLC), held in Reston, Virginia.

I don't know how many of you have ever experienced a significant earthquake. I don't mean merely being awakened at night, or something like that. I grew up in Mexico City, and I had my fair share of earthquakes. And it's actually very interesting: If you get woken up at night, that's one thing. But what happens if it hits during the day and you're wide awake? The earth moves under your feet; nothing seems to work; things move in crazy directions. And you feel a profound sense of disorientation.

It's a well-known fact that people can suffer extreme psychological disorientation--anxiety, even hysteria--from earthquakes. And this is not something that goes on only during the period of the earthquake itself, whether it lasts 15 seconds, or a minute, or two minutes, or even in the aftershocks. In serious earthquakes, this can affect you for months and perhaps for years. Populations--entire populations--are thrown out of whack, psychologically out of whack, by these types of earthquakes.

What we are facing in the world financial system today, is that kind of tectonic change: where nothing works the way it used to; where we are witnessing phenomena that have most people totally disoriented; where we are facing the kind of power that can level a building, level a city, level a civilization in a matter of moments.

In "The Road to Recovery" [EIR, Feb. 19, 1999], Lyndon LaRouche discussed this process, using the example that the rules that govern the functioning of water during normal periods of time are changing, because the water is becoming ice. We're in such a phase change today; we're in a period of transition where the old rules simply don't work. And the key issue is, that we have to know how to think--how to understand the process that's going on--in such a period of crisis. Because, as LaRouche was explaining yesterday, the phase changes happening today are characteristic of human development in general, even in the supposed "quiet times."

The economic earthquake that is now going on, that we are living through and are the middle of now, is also producing chaos--in case you've wondered why people are running around looking so disoriented, so anxiety-ridden, so hysterical. It's exactly the same type of process. And this can just as easily unleash mass hysteria in populations, as it can provide the opportunity for a solution. Needless to say, the purpose of our gathering here today, and the function of this movement, is to provide that solution, and to make sure that humanity has safe passage to the other side of the crisis.

There is, of course, a solution, which we've discussed in general terms here already, emphasizing the Common Good, or the General Welfare, of a society or of mankind as a whole. It's a concept of providing an answer, in economics, that will actually bring together, as opposed to separate into different competing forces, the different productive forces in a society, be they farmers and labor, consumers and producers, etc.

This is the same issue that is posed internationally. What is the common interest, what is the General Welfare that exists between, for example, countries like Mexico and the United States? Or, the same for Africa, say vis-à-vis the European countries, and so on.

How do we actually, scientifically, know what that General Welfare is? How can we describe it in more than a simply intuitive way? How can we hit that nail on the head?

The answer involves a concept which is elementary, but not simple, as LaRouche has stressed. It is not simple, and it is certainly not simplistic. It is going to take some work, some thinking here today, to discuss it.

Because the real issue that is posed is: how do you measure actual economic success? What is your metric? What are your units? What do you measure, to know if you are succeeding economically or not? How do you know that there can, in fact, be a common interest between labor and farmers, or between workers in the Third World and consumers in the United States?

This issue is posed concretely for us by the question of farm parity pricing in the United States, for example. How do you actually measure what the proper price, or value, of agricultural products should be?

If you look at it from the standpoint of the simple consumer, the argument is: "Well, the lower the price the better, because if you lower it further, people will be able to consume more with their meager resources." But that, of course, is not going to allow for the development of agriculture.

So, do you instead raise the price of farm goods sky-high? Well, that doesn't work either. So how can you decide? What's your metric? How do you measure this?

And what about the question of raw materials, such as oil? Is it in the interest of the United States to have extremely low prices for oil? What if that means the destruction of oil-producing nations like Venezuela, or Mexico, or Nigeria?

Or what about cheap steel? Do we want really cheap steel in the United States, as the proponents of NAFTA argue? After all, we could build factories more cheaply that way--right?

Or what about the question of Africa? "Isn't it time that we stopped spending so much of our good money on those wasteful people over there? We give out too much foreign aid, don't we?" Isn't that what we're often told? And it is just this kind of moral indifferentism--which is actually moral stupidity, which is criminal stupidity--which is seen in the general behavior towards a continent like Africa. And it comes from an underlying misunderstanding, a lack of a proper definition of what it is that is the metric of economic development: How do you know what economic progress is, and how do you establish a metric that can provide a scientific basis for achieving the General Welfare, not only for this nation, but for a community of nations--a community of principle--emphatically including Africa?

Now, in my remarks today, I want to address this problem from two standpoints. I want to get at the issue of metric--of the science of defining the General Welfare--from two directions. First, I'll discuss bankers' arithmetic, which is one way of counting, with which some of you may be familiar. We will look at that in terms of the current economic situation, especially as it relates to Brazil and the world financial crisis.

Second, I will take a little bit more time, and ask you to concentrate a little more intensely than many of us are perhaps accustomed to, on the issue of human arithmetic. And I want to do this with the aid of the great Renaissance mind, Cardinal Nicolaus of Cusa, who has already been discussed significantly at this conference, but who bears much, much further discussion, as one of the richest scientific minds humanity has ever produced.

Cusa's discoveries in science are actually the basis for solving this problem. In fact, in "The Road to Recovery," LaRouche says the following:

"Cusa's central discovery of later crucial importance for the development of modern economic science, lay in the realm of scientific method: how to use measurement as a way of indirectly, by negation, establishing the existence and nature of physical-scientific principles."

And we can add that this, in turn, lays the basis for establishing the existence of a way of knowing what the Common Good, or the General Welfare, is.

The world financial system: RIP

So let's first take up the question of bankers' arithmetic.

The last ICLC/Schiller Institute conference, held at the end of August 1998, followed by only a week or two what was probably one of the most crucial turning points in recent history: the de facto declaration of bankruptcy of the current world financial system when, on Aug. 17, 1998, Russia's Kiriyenko government declared a debt moratorium on their government domestic bonds (GKOs), and also announced various payment delays and moratoria on categories of foreign debt.

With this, the Russian government was declaring the de facto bankruptcy of Russia. The Russian state had gone bankrupt--whether it was stated that way publicly or not, whether in those words or not, that is in fact what happened. And it was announced to the world on Aug. 17, 1998.

From that point forward, two completely distinct processes got under way, in a visible form in the world.

On the one hand, you have had the increasingly hysterical, desperate efforts of bankers, oligarchs, and others to defend their dying system. They are intent on holding this together at all costs, and they say: "The only thing that matters is keeping the debt intact, sacrosanct, and paid. And we will do anything. We will hyperinflate; we'll slam on the brakes; we'll hit the accelerator; we'll put on the brakes again. We'll do whatever it takes to hold the system together." And they ultimately came down with a massively hyperinflationary program, as we'll discuss.

But at the same time, there was a veering-away of another alternative, of another option, which is what LaRouche has been calling the Survivors' Club. Because before then, the Survivors' Club was pretty much limited to China, which was the only country that had said "No, no. We won't go down with this system. We're not going to go there."

But then on Aug. 17, the Russians said "No, not for us either." And especially a couple of weeks later, when Primakov defeated Gore's buddy Chernomyrdin for becoming Prime Minister of Russia, Russia solidly joined the Survivors' Club. And then Malaysia joined on Sept. 1, with Prime Minister Mahathir's announcement of capital controls. And increasingly a new process was unleashed.

So you have a situation where, as of that date, you have, simultaneously co-existing two completely contradictory processes going on in the world, which cannot co-exist--not for long. On the one hand, there is a process that says that the debt, the credit system, the bankers' arithmetic must be kept intact, sacrosanct. It will not be touched except to make it grow further. And on the other hand, there are a number of governments, and political factions in other countries, which say: "No. People come first."

And that co-existence, as of water and ice, is what characterizes the global situation at this point.

Figure 1 gives you a quick idea of what happened in Russia on Aug. 17. The graph shows the interest payments that the Russian government was making on its GKO bonds, as a percentage of their total federal budget: The GKO payments rose sharply between 1995 and 1998, reaching up to 30% of their budget at the point they said, "No, no more, we just can't pay." And of course after Aug. 17, they went down to zero.

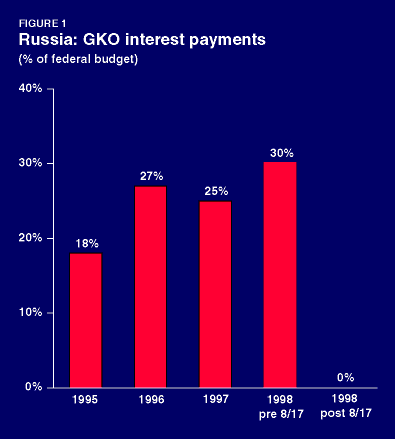
Not only the GKO debt but payments on the private foreign debt and bonds owed to the London Club were also frozen at that point. The government insists this is not a sovereign default, because they are willing to negotiate on this. For example, they are saying that, of the $17 billion in debt service due this year, they can pay $5 billion, but no more. They argue that they can't pay Soviet-era debt, in particular. And they also can't cover their debts to the International Monetary Fund (IMF) out of general revenues: If the IMF wants to loan Russia new money, they will use it to pay back older IMF debt, and nothing else. Otherwise, they don't want anything to do with the IMF.

The Russians have taken various other measures which are most significant, to get their productive economy going. They have dealt with the food crisis and the fuel crisis that they were facing, and although the problems are not over, they have addressed those issues.

The Primakov government has also addressed the domestic banking problem, where their policy has been to let the speculative banks sink or swim on their own, without a bailout from the government, while at the same time fostering national banking institutions to make the productive process work. They are in the process of creating an actual national development bank, and so on.

Furthermore, according to our best information, Russia right now is a hair's-breadth away from declaring capital and exchange controls as well--as deemed necessary by them for their survival.

So Aug. 17, 1998 was a real turning-point, where the world of globalization was handed its own death certificate. Where does that leave the world situation? To answer that, we have to start with a graph many of you are familiar with: LaRouche's famous "Typical Collapse Function" (Figure 2).

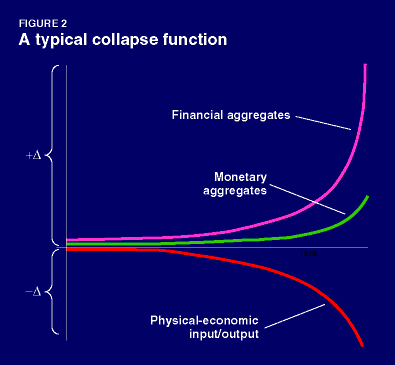
Please note: This is not three different functions; this is a function, a single function. The three curves are all integrally interrelated: one depends on the other. The uppermost curve shows the growth of financial aggregates, such as derivatives, which are growing completely out of whack, even beyond the growth of simple money supply (the middle curve). The bottom curve, the physical economic input-output, reflects a collapse of the physical economy which makes it impossible to continue to service those rapidly rising, hyperbolically rising financial obligations.

And of course what makes it all worse, is the connection between the curves: which is to say, that to feed the upper curve--the cancer--it destroys and further lowers the bottom curve. In other words, budgets are cut, wages are cut, austerity is imposed such that it contracts the very physical basis on which the financial aggregates exist to keep growing. So, you have a functional interrelationship.

Now, let's take a look at what this process looks like inside the United States. Figure 3 gives you a rough idea: These are real numbers. The Triple Curve was a heuristic device, a general representation of how the process works, that LaRouche developed a few years ago. But for the U.S. case we are presenting actual numbers of certain parameters. The uppermost curve shows the growth of financial derivatives held by United States banks at this point, to our best estimate: that comes in at close to $60 trillion in derivatives currently held by U.S. banks.

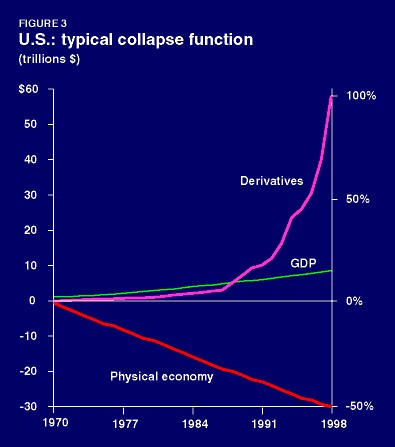
The middle curve is U.S. GDP. That's of course not the same thing as monetary supply, but it actually has a close relationship to it. GDP hasn't grown nearly as much as derivatives, but compare it to the physical economy, the bottom curve, which has been collapsing at the rate of about 2% per year, ever since 1967-70.

And that is the United States economy today.

Now, what do you do under these circumstances? Well, you can only go in one of two directions. On Sept. 14, 1998, President Clinton gave a speech at the Council on Foreign Relations (CFR), where he emphasized the need for a new financial architecture. We've got to do something to change this, he argued. We've got to stop the flow of speculative money flying all around the world. Clinton didn't provide a blueprint of how to do it, but he indicated the direction we have to go in, to stop the wild speculation.

But then the giant hedge fund, LTCM, collapsed on Sept. 23, about a week after Clinton's CFR speech. There were emergency meetings of the Federal Reserve, and Alan Greenspan lowered interest rates three times over a period of a couple of weeks, to hyperinflate the banking system back to life. At the same time, the political assault on the Presidency escalated, to weaken the President so that he couldn't address these issues effectively. Now, with the impeachment over, this places squarely on the center of the agenda again the issues pending from September 1998: Which way is the United States going to go? The way Wall Street says it should go? Or is it going to go the way LaRouche says it should go? That is the issue. Are we going to join the Survivors' Club, or are we going to be members of the Losers' Club--that go down with the world financial system?

Bankers' arithmetic

The latest development of the crisis, which brings us to the issue of bankers' arithmetic, has been the outbreak of a new financial crisis in Brazil.

Some of you may remember that LaRouche, in response to the outbreak of the crisis in the fall, issued five or six documents in a period of two or three weeks, which addressed what should be done: "Time to Tell the Truth," "People First!" and so on. In a lengthy essay written on Nov. 23, "When Economics Becomes Science" [EIR, Dec. 18, 1998], LaRouche also forecast that, in the beginning of 1999, within a period of approximately eight weeks, there would be a new outbreak of the world financial crisis which would be worse, bigger, and more explosive than anything that had been seen to date.

Many people asked themselves: "Where did he come up with eight weeks?"

Well, it's interesting, because that eight weeks would have taken you to Monday, Jan. 18. But on Wednesday, Jan. 13, seven weeks and two days after LaRouche made his forecast, Brazil blew apart, and devalued its currency, the real.

Why did it happen? Figure 4 shows Brazil's foreign exchange reserves, which up to the middle of 1998, had been as high as about $75 billion.

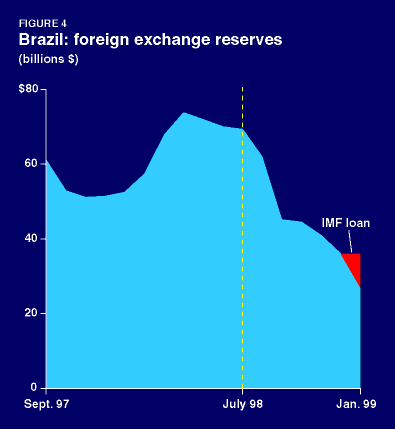
But then, when Russia blew out in August, the Brazilian reserves plummeted, as speculators--hedge funds and others--began to pull their funds out of Brazil. In fact, they pulled their hot money out of the entire so-called "emerging markets," fearing defaults across the board. As a result, Brazilian reserves began to plummet dramatically. There was a brief period where the IMF put together a hyperinflationary $41 billion bailout package, which didn't steady things for very long, and the reserves continued to plummet.

Now, the shaded triangle at the end of the curve is money which the IMF has recently pumped into Brazil--about $9 billion. Without that money, Brazil's reserves would now be about $25 billion--one-third the level they were at just six months ago. With that IMF money, they have just barely managed to hold things steady . . . for the moment.

In other words, bankers and hedge funds are pulling their money out of Brazil, and the IMF is throwing money in, to make sure that the bankers and the hedge funds can get out safely. And what of Brazil? "To hell with Brazil! Let it fall apart," say the speculators.

With its reserves plummetting in this fashion, Brazil on Jan. 13 was finally forced to devalue, by approximately 8%. But over the next 48 hours, a massive run against the currency developed. And the entire world financial system, in the case of LTCM, was hanging by a thread at that point.

There was an emergency meeting at the New York Federal Reserve once again, as there was in the LTCM case. George Soros was there, to help "encourage" people as to the direction to go, which was to bail out his hedge funds. There was significant discussion there, and in the subsequent Davos meeting of world financial leaders, of forcing Brazil to establish a currency board. A currency board amounts to bankers telling a country: "Don't you worry about a thing, honey. We'll take care of the money for you." That's what a currency board is: a British colonial system. "Now, don't you worry your pretty little head about this one little bit. We'll take care of everything, honey."

The way this works is that the bankers first obliterate your country: they create complete instability. And then they come in, and they offer a currency board as the only way that you can possibly regain stability. You just have to hand over control of your country--a minor cost--to the foreign bankers, who will run the currency board, and your entire monetary policy.

This is your typical mafia tactic. Late at night, they throw a chair through the window of your storefront, and then the next morning they come in and they offer you protection. "We've got a currency board for you. Wouldn't you like to try it?" they ask with a big smile on their face.

This is what the speculators did in Indonesia; this is what they did in Russia; and this is what they are now trying to do in Brazil. So watch out for this currency board business.

Now, what has happened in Brazil over the course of January? Let's look at this as if in slow motion, because this really shows you what bankers' arithmetic is all about. Brazil in early 1999 is like a slow-motion picture of bankers' arithmetic. So watch closely, but keep your hand on your wallet.

We've frequently talked about bankers' arithmetic, and many of you have seen this in our publications. Brazil is a good case study. Figure 5 shows Brazil's situation from 1980 to 1998. The solid line is the total official foreign debt of Brazil: In 1980, it was a mere $72 billion. Over the course of the next 18 years--from 1980 to 1998--Brazil paid $146 billion in interest payments alone. In other words, Brazil paid almost twice the original principal. And yet, at the end of that period, after paying in interest alone almost twice what they owed, they owed $231 billion--three times as much as the original debt.

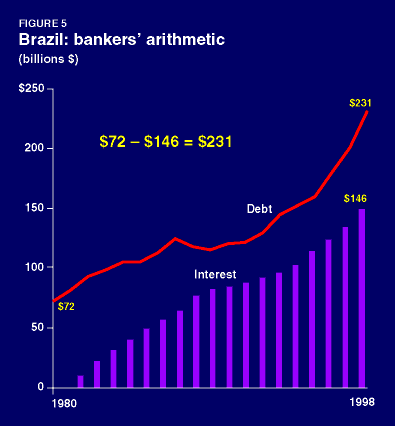
So, in bankers' arithmetic, 72-146=231. So that's bankers' arithmetic. That's how it works.

Now, some people have noted (and it's a valid point): "Well, wait a minute. You're not taking into account any new money that may have come into the country, and may explain the increase in debt owed; and you're also not including amortization payments--i.e., repayment of principal--which is an outflow."

That is true. This graph, this representation, abstracts from those two additional considerations. But when you take those two flows into account--any new, real money going in, and any principal repayment coming out--guess what? It's worse!

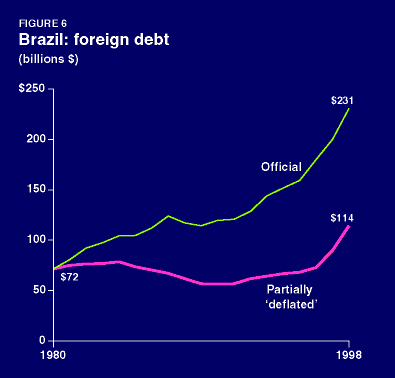
For example, I did a preliminary calculation, and in Figure 6 you can see what would have happened to Brazil's official foreign debt if it were "deflated" in this fashion (I used LaRouche's specifications on this point in "The Road to Recovery"). I emphasize that this calculation only partially "deflates" the debt, removing just one of its illegitimate components. To derive what is only legitimate debt, you'd have to deflate that even further, in ways I'll explain in a moment. But this gives you a rough idea of what the process is: The foreign debt wouldn't have grown to $231 billion in 1998; it would have only grown to $114 billion.

Okay, now what happened? Well, first of all, the official foreign debt is not really the full, real foreign debt of Brazil (see Figure 7). Because, in addition to the official foreign debt, you have to also consider government bonds which are denominated in dollars, and which are therefore de facto foreign obligations. You also have to count investment by foreigners in the stock market. And there are other similar kinds of foreign obligations, left out of the official foreign debt statistics.

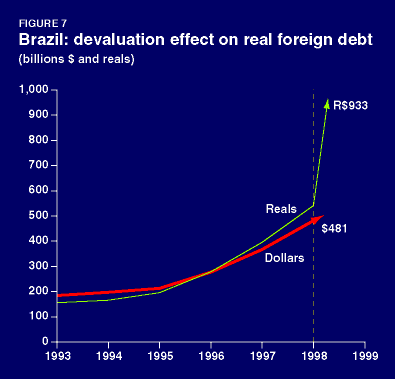
Taking all of this into account, we calculate that Brazil's real foreign debt at the end of 1998 was $481 billion.

Cheating, as taught at Harvard

Well, what happened in January 1999? The value of the real, the Brazilian currency, plummeted from 1.22 reals to the dollar in early January, to 1.94 reals to the dollar at the end of the month--about a 40% devaluation in one month's time.

Now, please take a seat as a Brazilian for a moment, not as an American. If you're a Brazilian looking at your foreign debt of $481 billion, back on Jan. 1 of this year, that $481 billion was equal to about 500 billion reals.

But a month later, after the 40% devaluation, that same $481 billion obligation is going to cost you 933 billion reals to repay. Bankers' arithmetic! And that is what you have to pay, as Brazil. The banks lend to you in dollars; but when you want to go back and pay at the original exchange rate, they say: "Oh, no. I'm sorry. Don't you know? The real has been devalued."

"What do you mean, the real has been devalued?" you reply, irate. "How could that be? The real can't be devalued, just like that."

And they say: "Of course it can. Don't you understand? Markets rule the world. You obviously have not studied economics at Harvard."

And there you are, not having studied at Harvard, and your foreign debt has just nearly doubled.

Now, this is only one of the ways in which bankers' arithmetic works. But before proceeding, let me just make this point clear. The way bankers' arithmetic works, the reason that this thing doesn't add up, and that you get this crazy arithmetic, is because they cheat!

How do they cheat? They change the unit of account on you. They lend you 100, and then they say: "Oh, no, I'm sorry. That 100 is now not worth 100 of your currency; it's worth 200. You've got to pay me 200."

Why? "Because we run the markets. Tough luck. What's the matter, buddy? Didn't you study economics at Harvard?"

So it's cheating based on the question of the unit of account--how you measure. It's a question of metric. So this first aspect of bankers' arithmetic is due to the devaluation effect.

Now, let's look at another component: the interest rate effect. For this, too, you can thank the bankers and the IMF. Back in January, the Brazilian government didn't fully follow IMF directions. Before the crisis, Brazil's interest rate was 25%. When the crisis hit, the IMF wanted them to raise interest rates to 70%. But so far, they haven't done that: They have "only" raised them to about 40%. [In the three weeks since this speech was delivered, the government hiked interest rates even further to 45%--DNS.]

But by raising domestic interest rates from 25 to 40%, the treasury bonds which the government had issued prior to that, changed in terms of what they were costing the government. Why? Because the government had done two very clever things. First, they issued some bonds denominated in dollars. Why did they issue bonds denominated in dollars? Because they were trying to convince "the market" that they would never, ever, ever devalue the real. Because no government would be so stupid as to issue bonds in dollars, and then devalue their own currency--right? And therefore, they were out to convince the markets that, since nobody could possibly be that stupid, that foreign speculators should have confidence in Brazil since there would be no devaluation.

So they issued 20% of their bonds in dollars . . . and then they were forced to devalue. So that raised the total amount of their foreign obligations, as per the devaluation effect.

But they also issued bonds, not at a fixed interest rate, but at a floating interest rate. Why? Again, to encourage "the market," to convince the market that they would never, ever be so foolish as to issue such bonds if they intended to dramatically raise interest rates. But then they were forced to dramatically raise interest rates. And the effect of this on their bonded debt, over the period of the first six months of 1999, is going to raise that debt from about 320 billion reals--where it is today--up to close to 500 billion reals in six months' time (see Figure 8).

Over the period from December 1995 to December 1997, Brazilian treasury bonds outstanding were growing "merely" at 40% a year. Over the next year, they grew at "only" 50% a year. Now, they are going to grow at 120% a year. Again, bankers' arithmetic.

So much for the devaluation effect, and the interest rate effect.

The third effect that I want to talk about, is the impact of changing terms of trade, which is yet another way the bankers cheat. They change what they pay you for your exports, if you're a Third World country. They lower those prices, and they raise the prices of what you import. So that the price of what you are exporting, with which you pay your foreign debt, drops.

Again, they're changing the unit of measurement on you in mid-stream. When you complain, and say: "Hey, wait a minute! That's not fair," they shoot back: "Nobody ever said it was fair. That's the market. What's the matter? Didn't you study economics at Harvard?"

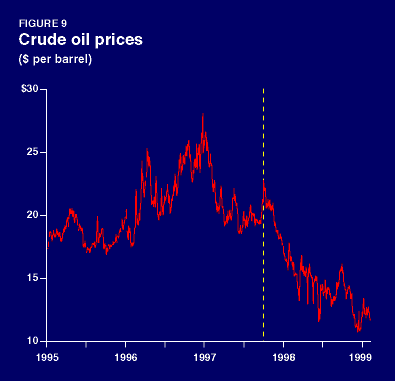
As an example, look at what happened to the price of oil over the last eight or nine months: It fell by half (see Figure 9). What if you are Nigeria? What if you are Venezuela? Oil dropped from $22 per barrel down to $11 per barrel. What if you are Mexico, and 40% of your government budget revenue comes from oil? Well, in that case, you just had 20% of your total budget wiped out. If 40% of your budget is oil, and the price falls to half, you just lost about 20% of your budget. What this translated into, for Mexico, is that its public debt became de facto that much more expensive to service, just as a result of the falling price of oil (see Figure 10).

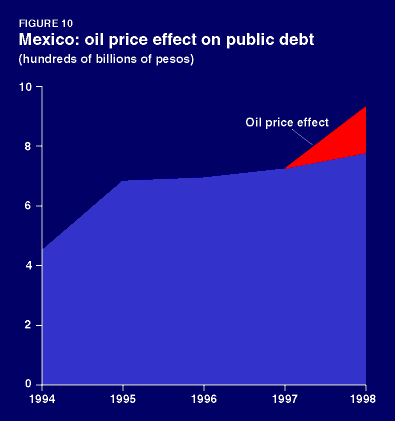
The debt was already rising, but the oil price effect raised its real internal cost to the physical economy that much further. And if you're a Mexican, you watch in shock as your country has no budget for infrastructure projects, no budget for health, no budget for food subsidies, no budget for anything--except for paying the debt. And you say: "Hey! That's not fair!" And George Soros leers back at you: "Ha. You obviously didn't study economics at Harvard, or Princeton. But don't worry, we'll appoint a good adviser to you who did. We'll send you Arminio Fraga."

So these are three ways in which bankers' arithmetic actually works. It's cheating. It's cheating because they control the game, they control the metric. They say, "This is the unit of account, this is how we're going to count everything." And if you stick to that, if you say, "Yes, prices, money, debt, this is all sacrosanct, and the real world be damned," then the world will be damned, and we will have a New Dark Age.

There is no way this system can co-exist with human beings. It can't happen any more. The water is turning to ice.

You know those cartoons, or drawings, where they show you two pictures, and there's one that's slightly different from the other and they ask you, "What's wrong with this picture?" Well, in this case, everything is wrong with this picture! There's nothing right; the whole financial system is completely wrong. It has to be completely, totally, 100% changed.

Because under these conditions you cannot establish a principle of General Welfare, you cannot establish the Common Good. There is no basis on which you can have a community of principle among sovereign nations. You are going to have a global Dark Age.

Human arithmetic

How do we deal with this? What is the alternative? I think nearly everyone here has a pretty good smell that this system doesn't work. But what does work? If the IMF system is wrong, what's right?

You might say: "People come first, population comes first. That's the most important question." That's true enough, but how do you achieve that? How do you measure success? In fact, how do you count at all? How do you actually count in an economy? How do you measure things?

If you say: "One, two, three, . . . ." the question back is: One what? Two whats? What are you measuring, and what is your unit of measurement? You say, "Okay, we'll measure things in dollars."

Well, that obviously isn't going to work, because money, like credit, is a political fiction, and accounting in those terms is not going to work, for the reasons that we saw with bankers' arithmetic.

So having looked at some of what LaRouche has to say about physical economy, you might say: "Okay, let's measure physical output."

Well, if we measure the economy in tons, or something of the sort, that might be a little more physical, but it's not a whole lot smarter than using money as your metric, because one ton is not the same as another ton. For example, you could have a ton of gambling chits in Las Vegas, or you could have a ton of steel. So simple tonnage obviously is not going to do the trick.

Then you might try something a little more sophisticated, such as a market basket of products, for example, a market basket of consumer goods. And you lay out the physical items required for that market basket, and for the inputs that go into each of those, and you conclude, "Good; that's what we're going to use."

But there is still a fundamental epistemological problem here, which is that things change. Things change. And there is no fixed yardstick, even a physical one, that you can actually use to measure in an economy. Because if you use a fixed yardstick, but the economic surface that you're measuring changes--the way a curve moves, or the way a rubber band stretches, or anything of that sort--if your surface changes, a fixed yardstick is simply not going to apply equally in different portions of your surface, nor over time, in Period Two as it did in Period One.

LaRouche states the issue directly in "The Road to Recovery":

"The intrinsic values in economic processes have no scalar (e.g., linear) measure, no simple yardstick. Everything about human life in this universe is to be measured against a specifically non-linear standard, an anti-entropic standard of change."

LaRouche elaborates on this concept in his reference to the phase-changes from water to ice. In order to figure out how to count in an economy, he explains, you must ask:

"What is the principle of action, the principle of change which we should employ to craft an unchanging definition of water which is equally appropriate for each and all physical states and also the transitions among those possible states?"

In other words, if the rules of water don't apply to ice, how can you define a principle of action, and a measurement of that principle of action, that applies equally to both of the states--water and ice--and most importantly to the transition between those physical states?

Now, posed this way, this gets us back to a very old, very elementary, yet very profound philosophical question: What is it that does not change throughout all change? If everything around us in the world is changing, what is it that does not change?

This is Heraclitus's famous example of the river: How can you possibly step in the same river twice? Because, if you step in a river in one instant, and then you step in it again, one instant later, can you actually legitimately say it is the same river?

Everything about it has changed, right? The water has moved, the pebbles have moved, the shoreline has moved, albeit a little bit. The wind has moved, the trees have moved--everything is different. How can you say it's the same river?

In fact, anyone who is operating on the basis of sense-perception and linear scalar measurements, cannot possibly argue, legitimately, that it's the same river, because it has completely changed.

Well, what about the question of simple identity--saying "I," the word "I"? What is "I"?

Is "I" the perceptions you have of yourself at the moment you begin to utter that one-syllable word? Or is it the perceptions you have of yourself when you have finished uttering that one-syllable word--even if you say it real quickly. No matter how fast you utter it . . . sorry, you changed. Everything changed. Nothing is the same. Every single particle is in motion. So what in the world are you talking about?

And of course the existentialists and other lunatics in fact conclude: "Oh, you don't exist. I don't exist." Pure skepticism: There's nothing that can be known, they argue, we just have sense perceptions, and they are all around us, like a kaleidoscope.

Now, Plato addresses this issue in the following manner. Plato argues that there are a couple of different ways to look at this problem. You can look at a series of items in a set. For example, let's take the idea of a box (see Figure 11). Now, you can have a whole set of boxes: box₁, box₂, and so on. And you can have an infinite number of such boxes.

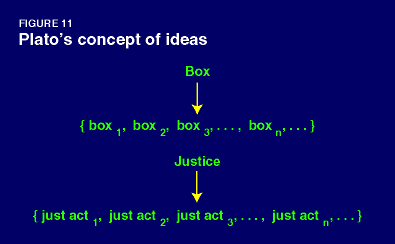
But there is no possible way that you can add up all of those different specific boxes, to reach the concept of Box. Because when I say "Box," you each have an idea in your head, which is a single unified concept. It's none of the specific boxes, right? And yet it is a definite idea.

In fact, you know if a specific box is indeed a Box, to the degree to which it does or it does not correspond to your concept of Box, your idea of Box, or what Plato sometimes call the form, or Cusa later talks about as the exemplar.

Plato's argument is very straightforward, very simple. He says the form, the idea of "Box," has a higher order of existence and is more real than any of the specific boxes. And he argues, most significantly, that this idea cannot be derived from any summation of all of the individual boxes. No matter how long you extend that process, or that set, it is still not going to give you the concept of "box."

The same thing with Justice. If I say to you, "That was a just act," you have some idea of what I'm talking about. But the specific just act is not the same as the concept of Justice. And again there is no compendium, no summation of particular, individual just acts, no matter how many of them you think of, no matter how long you continue--ad infinitum and ad nauseam both--you will not come to the concept of Justice.

The concept of Justice is prior. The concept of Box is prior to the individual boxes. And they are of a completely different nature: They are incommensurable. They cannot be measured with the same yardstick.

The reason is that there is a fundamental distinction between the cause of a set of items, and the internal contents of the set itelf, a difference between the cause and the particulars which that cause produces. This is the way the universe is organized, and it is the way the human mind is organized.

Furthermore, the cause is of a higher order of ontological existence of reality, than the specific manifestations it produces.

Incidentally, all of the famous ontological paradoxes in philosophy are of this form. You may be familiar with some of them. For example, I want you to tell me if the following sentence, which I'm about to utter, is true or false: "This sentence is false."

Okay, is that sentence true or is it false?

Well, if it's true, then what it says is true, which is that it's false. But if it's false, then its content cannot be true, which means that it isn't false. So if it's true, it's false; and if it's false. it's true.

Now, that's not just a word game. That's an ontological paradox. Why? Because you're trying to force the mind to mix two completely different levels of existence--the cause of a set and the internal contents of a set--and measure them with the same yardstick. But the cause of a statement and the contents of a statement are incommensurable, one with the other. Thus, the paradox.

Cusa: measuring the incommensurable

This now brings us to the issue of Nicolaus of Cusa's discovery.

I want to look at two essays by Cusa. One is On Conjectures, which was written in 1440, the same year in which he wrote his masterpiece, On Learned Ignorance, and it is to some degree a shorter companion piece of that.

Cusa explains that the way the mind works, is that it has four levels of unities which it understands (see Figure 12).

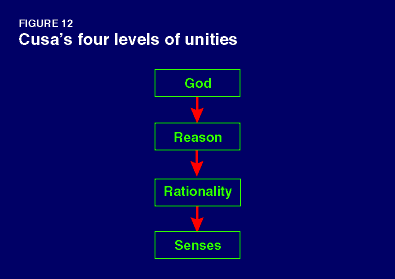
At the highest level, we have God. Below that, we have Reason, which he sometimes calls Intelligence. Below that is Rationality, which is sort of formal-mathematical or linear thought. And then below that is Body, or the Senses.

Cusa says that the first of these--God--is the unity of all, and the cause of everything beneath it. He is also the standard by which you measure everything which He has created. In other words, you measure that which is created by that which creates it. It is the infinite which measures the finite--only so.

Think back to Plato and his idea of the sets. It is only the concept, the unifying concept of the set, which provides measurement for all of the contents within it.

So, God is the highest--the unity of all.

The second level is the level of Reason, or Intelligence, in which the human mind is capable of generating actual concepts that unify what would otherwise seem to be contradictory elements or thoughts--coincidentia oppositorum--which Aristoteleans deny can exist. But it is the ability of the mind to form a unified idea out of things which don't seem to come together in exactly the right way--like a metaphor, or a joke, or an ambivalence. In all such cases, it is a concept in the mind, which is not in the elements which compose it, but is in the mental action which conceives of the two seemingly contradictory elements as a unified whole.

The third level--Rationality, or formal thinking--exists only because it is illuminated by Reason. Rationality, Cusa explains, is the level at which you put a name, a label, on something. You say: "Oh, it's this, this is what it is called, that is its name."

In On Conjectures, Cusa writes:

"For if rationality investigates intelligence, which it comprehends with no sensible signs, how could it begin this investigation if the light of intelligence did not incite its illumination? Intelligence is therefore related to rationality, as God Himself is to intelligence."

And again: "Intelligence is therefore nothing of that which can be said or named; but rather is the origin of the rational concepts of everything, just as God is the origin of intelligence."

Aha! Now that's interesting: Intelligence, or reason, is related to rationality, as God is related to reason.

As for the fourth, lowest level, that of sense-perception, Cusa says that, ironically enough, even to perceive something requires the activity of rationality in order to distinguish among the different perceptions. In other words, there is no such thing as sense-perception prior to the activity of mind to decide where perception begins and ends--to delimit it. Recall the case of the river that we were talking about before. The concept of "river" is clearly not simply a series of perceptions. There is something else that is going on. Cusa discusses it as follows:

"The senses perceive and do not distinguish. Every distinction is indeed from rationality. So rationality makes use of the senses as an instrument, in order to distinguish the sensible. But it is rationality itself which in the senses distinguishes the sensible."

So you can't even have sense-perception without a prior, higher-order function of mind, which is rationality, to distinguish between those perceptions.

Here is where the fun really starts. Because Cusa then says that these four levels are incommensurable. You cannot apply the same metric to them, because they don't exist in the same type of geometry. They co-exist in the same universe, of course, but they don't have the same metrics. They cannot be counted in the same way. You are talking about incommensurable types of mental activity: Senses, Rationality, Reason, and God.

Cusa puts it this way:

"One world does not count or speak or do something as another does; for example, intelligences are not counted as stones or animals, they also do not speak as men; but rather each world employs its own manner."

And then he adds an argument which appears quite frequently in Cusa, and which you may have heard about: that these two kinds of thinking are as fundamentally distinct, incommensurable, as are a polygon and a circle.

"Hence, a rectilinear polygon is improportionate to the circle, because rationality does not attain the coincidence of the curve and the straight line."

In other words, in the same way that a polygon and a circle are incommensurable--that is to say, there is no number of angles, or sides, that you can add to a polygon to eventually get to a circle, because you are talking about different species, and there is a change that goes on--so, too, with the four different levels of unity of the human mind, and what each is capable of thinking.

Now, if they're incommensurable, how do you count? And if this is how an economy work (which I think you might guess is what I'm driving at), and an economy moves from one technological phase to a higher phase to a higher phase, how do you count across those changes? How do you count between water and ice? How do you change from one technological mode to the other?

With the problem so posed, let us now delve into the essentials of Cusa's solution to this problem. Because it provides the elementary, if not simple, answer to the question of how do you identify, and how do you generate the General Welfare, or the Common Good.

Mind is the metric of the universe

If you can't count with the same unit of account across phase changes, what do you do? In other words, what is the causality of the connection across the phase change?

In a dialogue which Cusa wrote in 1450, called The Layman: About Mind, he explains that the infinite--the relatively higher order existence--measures the finite. You cannot measure the infinite with the finite; you can only measure the finite with the infinite. The way the human mind works, what it is in essence, is that it measures. It does this by embracing within itself the idea, or the exemplar, which produces all of the specific predicated things which occur in the sensible world. So the mind functions as a relative infinite to measure that which is merely finite, against itself."

Cusa says:

"Mind is that from which comes the limit and measure of all things. In fact, I propose that `mind' (mens) is so called from `measuring' (mensurare). . . . Mind [is] the power in us which embraces conceptually the exemplars of all things."

Cusa explains that mind does not work at the level of the merely sensible. It is not a matter of perceiving things, of cataloguing those sense-perceptions, and then naming them. Because that which we name, and we call a specific thing, does not come from our perceptions, but it comes from the prior activity of mind--the prior activity of reason--which allows us to form a single concept, a unity; to understand that unity, which does not lie in its perceived predicates; and only then to bring it down to the level of naming it.

Cusa says:

"Something is present to mental intuition [reason] which was not present to sense nor to [rationality], namely the exemplary and incommunicable truth of the forms which are reflected in sensible things. . . . Hence, genus and species, insofar as they are matters of naming, are mental constructs which human reason has made for itself."

Cusa goes on to attack Aristotle on this point, for arguing the opposite. Cusa notes that the Aristoteleans say that "to understand is an accident." In other words, the Aristoteleans rule out completely what Cusa says is the primary activity of mind.

Cusa's punchline on this subject, is that this activity of mind is what gives the human being life, and it therefore is his soul. This is what a soul is, according to Cardinal Nicolaus of Cusa: the activity of reason of the human mind. It is this which makes man in the living image of God; this is what imago Dei means.

"Mind is a living substance. . . . Its function in this body is to give it life and because of this it is called soul. Mind is a substantial form or power."

And further:

"Our mind is the image of that infinite being which enfolds all images. . . . Knowledge of God, His `face,' is accessible only in mental reality whose object is truth. It is not further accessible except through mind so that mind may be the image of God and of all God's images following upon the exemplar itself."

Please keep in mind this metaphor of "God's face."

Now we have come to the core of the matter, to wit: mental activity in conceiving of new creative thoughts is that which distinguishes man from all other species, and makes man in the living image of God.

In another Cusa dialogue, called The Layman: About Wisdom (1450), one of the participants in the dialogue, the Orator, asks the wise Layman:

"I want you to tell me how I am to form a concept of God, since he is greater than can be conceived."

And the Layman responds: "You may do so just as you form a concept of a concept."

Cusa is telling us that the way you form a concept of "God," is in the same way that your mind forms a concept of "concept." In other words, if you study the way your own mind works, you understand--negatively reflected--precisely that quality in yourself which is in the image of God. And that is how you know God--as reflected in yourself, and all human beings, through that creative power.

This relationship, this way in which the infinite is the metric and standard by which to measure the finite, is elaborated in this same dialogue by Cusa, with the following beautiful metaphor about the human face:

"Absolute Justice and Absolute Goodness enfolds all exemplifiable things. . . . It enfolds these much more perfectly than your face enfolds all the images that are formable of it. . . . For all possible depictions of your face are precise, correct, and true to the extent that they partake of, and imitate, the form of your living face."

So the living face, the moving face, the one that is alive, is the standard by which you measure any particular depictions of it.

It is exactly this concept which provides the basis for answering the question of what human arithmetic is: Man's mind is actively involved in knowing the universe, by "measuring" it against his own creative activity. In The Layman: About Mind, Cusa says:

"Mind is a living measure which achieves its own capacity by measuring other things."

Mind is not of the nature of changeable things, which are grasped by sense-perception, but of unchangeable things, which it discovers in itself.

So true science is subjective. You know the world by changing it through mental activity, through ideas, through creativity. And there is a demonstrable coherence between the process of your own mind's working and the physical universe as it is actually organized. As LaRouche has put this point so incisively, we know this to be true, because when you do the right thing and think creatively, the physical universe "obeys" you, and responds accordingly. So you know that the two--man's mind, and the physical universe--are organized in the same way.

Where does all of this leave us, on the issue of human arithmetic? What have we shown?

1. Man's mind is characterized by concept formation, not perception.

2. Man's mind is coherent with the lawful composition of the universe, and it knows those laws through its own action.

3. In physical economy, there are a series of phase-changes which are incommensurable in terms of their internal metrics. So, classrooom mathematics does not work to provide a metric.

4. But each of these phases is produced, in an ordered succession, by a higher level cause, which is mental creative activity, whose agent is the human mind--the proper metric of human arithmetic.

5. Specific policies--taxes, tariffs, credit policy, and so on--are all to be measures as to the degree to which they fulfill the General Welfare so defined, and only so defined. No lesser standard will work.

6. One human mind communicates such creative ideas to another, only by recreating those concepts within the sovereign provinces of the other's mind.

LaRouche emphasizes this point regarding the communication of ideas as follows (and recall here Cusa's image of knowledge of the human face):

"The two minds in question will tend to generate a conception--a more or less distinct concept, analogous to the way in which the mind registers the identity of a person's active face--an identity ready to receive its proper christening with an appropriate name."

López Portillo: the Common Good

I want to close with quotations taken from the most recent video report issued by EIR, on the subject of the Eurasian Land-Bridge and Helga Zepp-LaRouche's recent trip to Mexico. The quotes are from former Mexican President José López Portillo. The first is from an October 1982 speech he delivered at the United Nations, about three or four months after meeting in Mexico City with Lyndon and Helga LaRouche. The second is from a speech of López Portillo's just last December, at the Mexican Society for Geography and Statistics, where he was the official commentator on Helga Zepp-LaRouche's keynote address.

As you read these words, I suggest you think about them from the standpoint that we have discussed here. Apply the concepts of Cusa regarding what we have called "human arithmetic," to look into the mind of López Portillo, and understand the necessary concept of the General Welfare--of the Common Good of humanity. And then from that standpoint, rethink the tasks we face today.

"But the most constant concern and activity of Mexico in the international arena, is the transition to a New Economic Order. . . .

"We developing countries do not want to be subjugated. We cannot paralyze our economies or plunge our peoples into greater misery in order to pay a debt on which servicing tripled without our participation or responsibility, and with terms that are imposed upon us. We countries of the South are about to run out of playing chips, and were we not able to stay in the game, it would end in defeat for everyone.

"I want to be emphatic: We countries of the South have not sinned against the world economy. Our efforts to grow, in order to conquer hunger, disease, ignorance, and dependency, have not caused the international crisis. . . .

"After major corrective efforts in economic affairs, my government decided to attack the evil at its root, and to extirpate it once and for all. There was obviously an inconsistency between internal development policies, and an erratic and restrictive international financial structure.

"A reasonable growth policy was irreconcilable with freedom to speculate in foreign exchange. That is why we established exchange controls.

`Given our 3,000 kilometer border with the United States, exchange controls can only function through a banking system that follows the policies of its country and government, and not its own speculative interests or the fluctuations of international financial chaos. That is why we nationalized the banks.

"We have been a living example of what occurs when an enormous, volatile, and speculative mass of capital goes all over the world in search of high interest rates, tax havens, and supposed political and exchange stability. It decapitalizes entire countries and leaves destruction in its wake. The world should be able to control this; it is inconceivable that we cannot find a formula that, without limiting necessary movements and flows, would permit regulation of a phenomenon that damages everyone. It is imperative that the New International Economic Order establish a link between refinancing the development of countries that suffer capital flight, and the capital that has fled. At least they should get the crumbs from their own bread. . . .

"The reduction of available credit for developing countries has serious implications, not only for the countries themselves, but also for production and employment in the industrial countries. Let us not continue in this vicious circle: it could be the beginning of a new medieval Dark Age, without the possiblity of a Renaissance. . . .

"We cannot fail. There is cause to be alarmist. Not only the heritage of civilization is at stake, but also the very survival of our children, of future generations and of the human species.

"Let us make what is reasonable possible. Let us recall the tragic conditions in which we created this Organization, and the hopes that were placed in it. The place is here, and the time is now.

And in December, 1998, former President López Portillo stated:

"I congratulate Doña Helga for these words, which impressed me, especially because first they trapped me in the Apocalypse, but then she showed me the staircase by which we can get to a promised land. Many thanks, Doña Helga.

"Doña Helga--and here I wish to congratulate her husband, Lyndon LaRouche. . . . It is now necessary for the world to listen to the wise words of Lyndon LaRouche. Now it is through the voice of his wife, as we have had the privilege of hearing. How important, that they enlighten us as to what is happening in the world, as to what will happen, and as to what can be corrected. How important, that somebody dedicates their time, their generosity, and their enthusiasm to that endeavor. . . .

"Thus the importance that someone in the world is thinking on behalf of everyone, and is opening doors. Let us hope, Doña Helga, that your husband can influence the government of the United States, so that the proposals which you so brilliantly have laid out to us, can, in some way, be realized, and with them, that each people can express its uniqueness in the cultural realm, and in every possible aspect. Thank you."